CSET Math Subtest II
Overview
Subtest II has 35 multiple-choice questions and 3 constructed-response questions.
There are two domains, both with specific competencies:
- Geometry
- Plane Euclidean Geometry
- Coordinate Geometry
- Three-Dimensional Geometry
- Transformational Geometry
- Probability and Statistics
This section tests your knowledge of geometric concepts related to the Euclidean plane, which is just a flat, two-dimensional “area” with no coordinates or any other defining characteristics. The simplest concrete example of a Euclidean plane is a plain sheet of paper. It is named after the mathematician Euclid, who outlined many of the facts (which he called axioms and postulates) related to points, line segments, rays, lines, angles, and other figures which can be constructed in the plane. Euclidean geometry can be done with only a straightedge and compass, and all of Euclid’s work in his book,
The Elements is based on constructing figures using just these two tools.
Let’s take a look at some of the common elements found in plane Euclidean geometry.
Points
The most basic unit of structure found in plane Euclidean geometry is the point. It is a single dot, usually used either to indicate a part or to build, some other figure. In geometry, we name things by the points that make them, so the point in the example below would be called point A.

Line Segments, Rays, and Lines
Line segments, rays, and lines are all defined by a pair of points in the Euclidean plane.
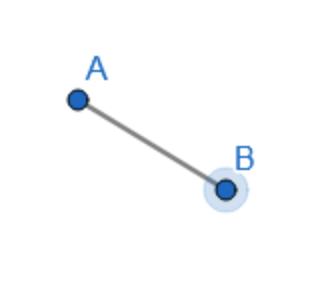
Let’s start simply. If you draw two points on a sheet of paper and connect them with a straightedge, without going past the points, you have created a line segment. Defined simply as a line connecting two points, this is the simplest linear form in geometry. Lines, rays, and segments are all named by the two points that define them. Pictured below is line segment AB:
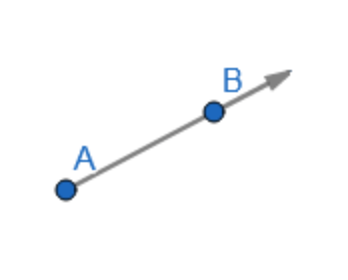
In order to draw a ray, begin with two points, draw a line from one point to the other, and then keep going. A ray begins at one point, passes through another, and then keeps going forever. This is denoted by placing an arrow point on one end of the ray. Pictured here is ray AB:
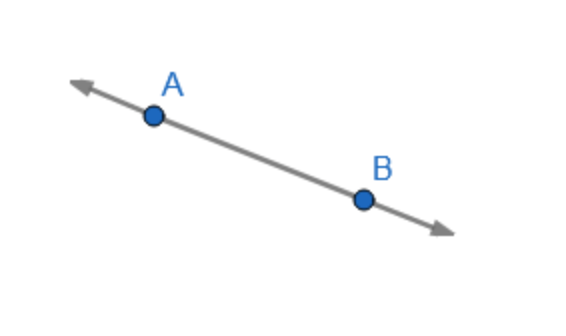
Finally, let’s draw a line. Just like with a line segment or ray, begin with two points. Then, draw a line that passes through both of them but keeps going on both sides – forever! This is denoted by placing arrow points at both ends of the line. Pictured here is line AB:
Angles
You can think of geometry, and a lot of math really, like a ladder. Each concept builds on the concepts before it. We started with a point, the most basic element in geometry. By adding a second point, we can create a line. Now, by creating a second line, we can create an angle.
To understand angles, let’s make one. First, draw a line by connecting two points and extending past them. Now, draw a second line that intersects the first one. A pair of lines, as long as they intersect, will always intersect at a point. We call this point the vertex, and at that point they make angles. Angles are named by their parts, with the vertex always in the middle. For example, pictured below are lines AB and CE, which intersect at point D :
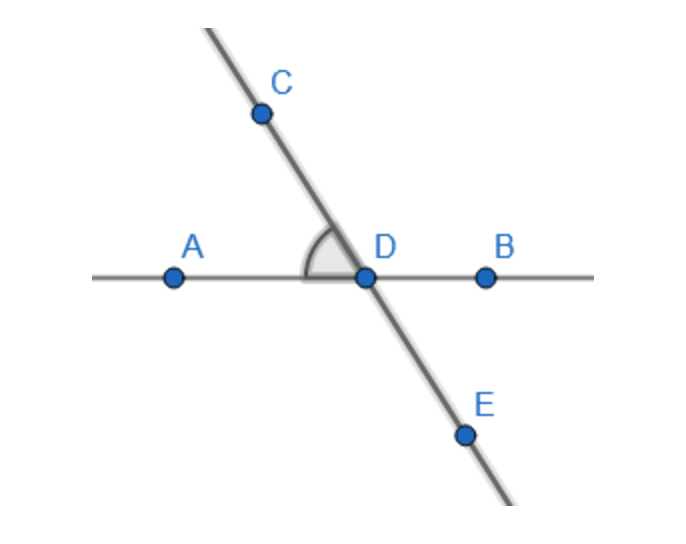
The indicated angle is angle ADC. Since the vertex is D, it is in the middle of the angle’s name. Sometimes, you may see this denoted with an angle symbol: ∠. For example, ∠ADC.
An angle that looks like a plain straight line is called a straight angle and measures 180 degrees. In the example above, the angle ADB is a straight angle.
An angle that looks like a square corner is called a right angle and has a measure of 90 degrees. An angle that is smaller than a right angle is called acute, and an angle that is larger than a right angle (but smaller than a straight angle) is called obtuse. In the example, angle ADC is acute, and angle ADE is obtuse. We denote right angles with a square indicator, instead of a curved one. See the right angle FGH in the example below:
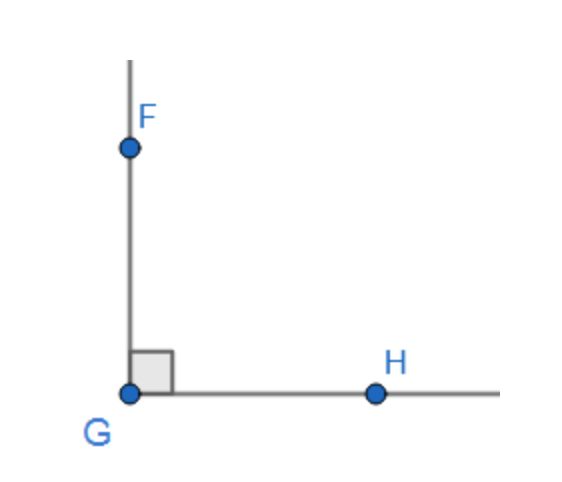
Two lines that intersect at right angles are called perpendicular, and two lines that never, ever intersect are called parallel (you can remember this, because the two L’s in the middle of “parallel” form parallel lines).
Geometry: Coordinate Geometry
So, let’s start with Plane Euclidean Geometry.
Geometry: Plane Euclidean Geometry
In-plane Euclidean geometry, we study points, lines, figures, and some of the relationships between those components; however, to get a little deeper understanding of those relationships, as well as discover some new ones, it is helpful to add some context. That is where coordinate geometry comes in.
Coordinate geometry is done on the coordinate plane, also known as the Cartesian plane. The coordinate plane is made up of two
axes (axes is the plural of axis), called the x-axis (horizontal) and the y-axis (vertical). Now, by using a system of ordered pairs of x and y coordinates, we cannot only draw points, lines, and figures, but we can determine their locations.
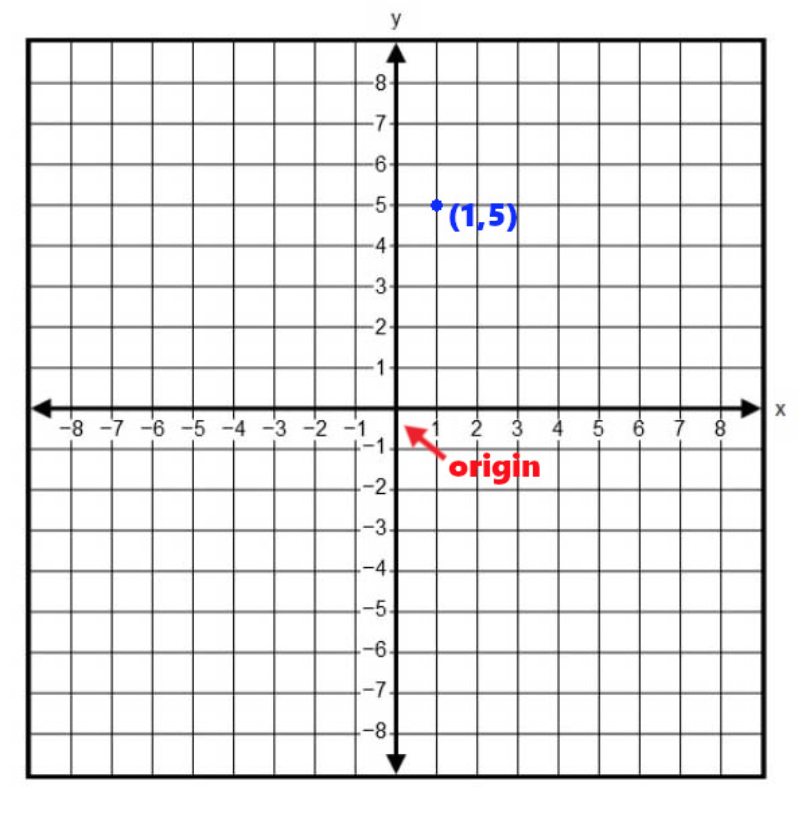
An ordered pair is like a set of instructions on how to get to a point on the coordinate plane. It looks like a pair of numbers – for example, (1,5); (3,0); (-5,1). These ordered pairs explain the location of a point in terms of the axes – (x,y) – first x, then y. You can think of it like getting directions from your navigation app: first, go this far along the x-axis, and then go this far along the y-axis.
When using ordered pairs, we always begin at the origin – the point (0,0), where the two axes meet. In the coordinate plane, the x-axis is positive toward the right and negative toward the left. Similarly, up is positive on the y-axis and down is negative. So, in order to plot the point (1,5), we will begin at the origin, go 1 to the right on the x-axis, and then go up 5.
In coordinate geometry, we have some important formulas to help us determine lines in the plane, the slope of a line, the midpoint of a line, and the distance from one point to another. They are:
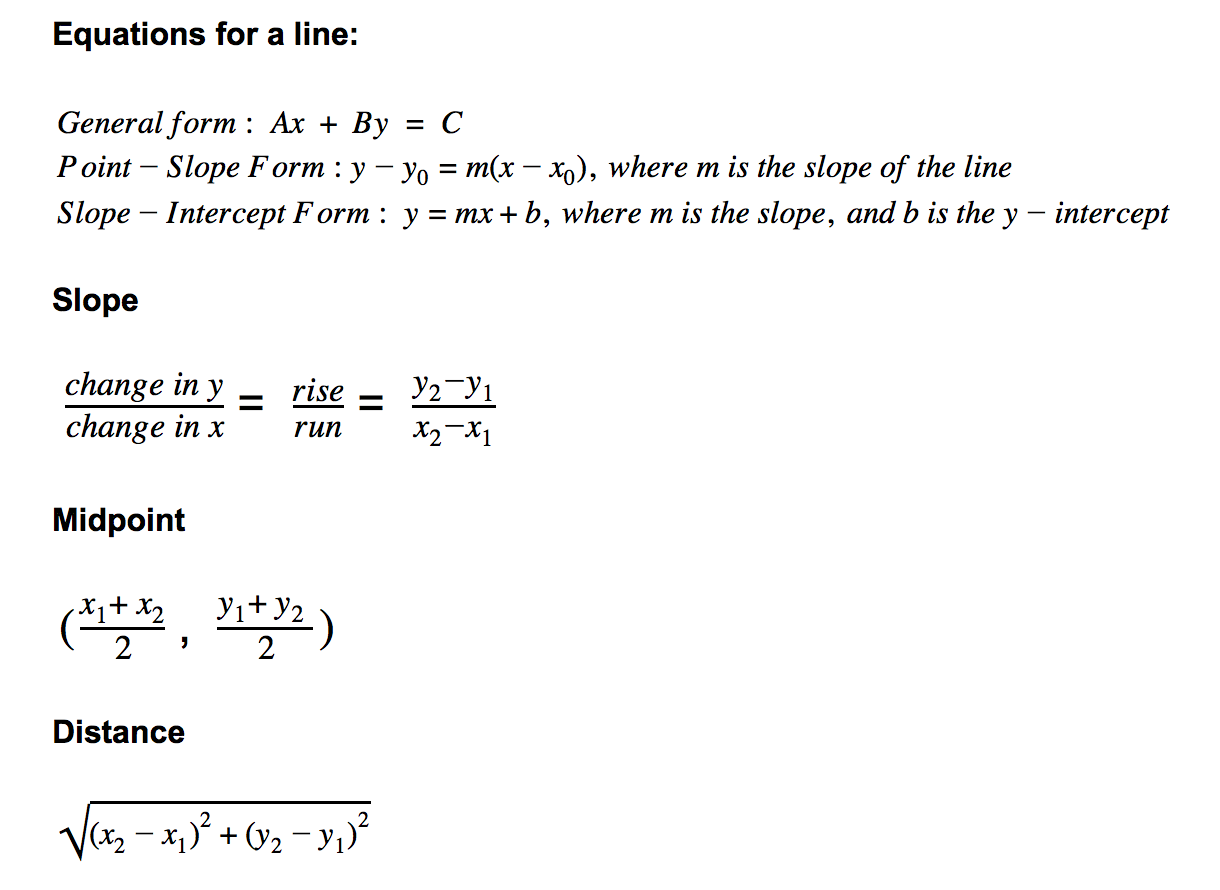
Geometry: Three-Dimensional Geometry
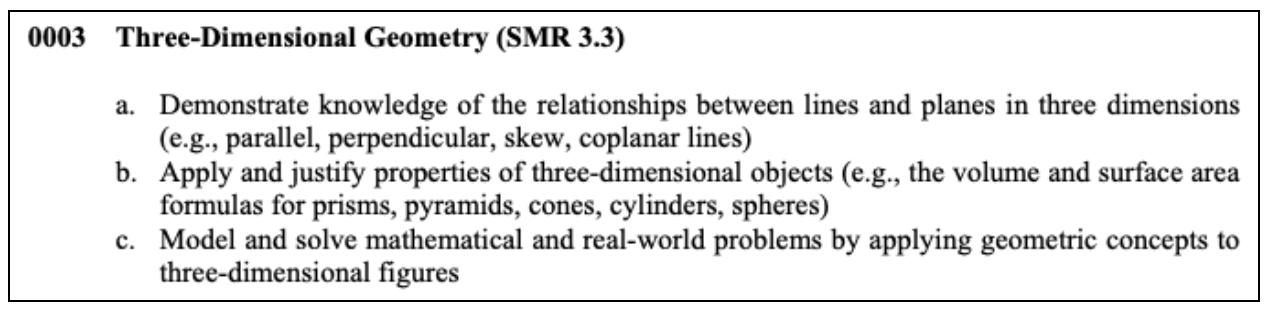
Three-dimensional geometry is, as the name would suggest, the mathematics of shapes and objects in three dimensions. It involves shapes like cubes, cuboids, and polyhedrons; 3D geometry is all about objects that have height, width, and depth. Just like 2D geometry can be done on a plane, which has two dimensions, 3D geometry adds a z-axis to the plane. You can visualize the z-axis as going through your screen or a sheet of paper when you look at a Cartesian plane. This is called a space, and in the case of three dimensions, it’s called a 3-space.
Shapes in three dimensions are called geometric solids.
They include:
- Cube: a solid whose faces are square.
- Cuboid: a solid whose faces are rectangular.
- Square Pyramid: a solid with a square shaped bottom and four triangular sides.
- Triangular Pyramid: a solid whose faces are all triangles.
- Cone: a solid with a circular face at the bottom and a single point at the top, called the apex; every line segment connects the apex to a point on the circle.
- Cylinder: a solid with circular faces connected by a curved surface.
- Sphere: a solid defined by a central point P and every point in three dimensions at a distance R from P; it looks like a ball.
Many 2D shapes have 3D solid analogs. For instance, a sphere is the 3D analog of a circle. We understand what these shapes look like, and thanks to geometry, we can describe them by simply extending plane geometry slightly.
In two dimensions, the parallel postulate tells us that when given a point A and a line BC which does not contain A, there exists one and only one line passing through A that does not intersect BC. This is not true in three dimensions! Once we add the third dimension, the z-axis, you can create all sorts of lines coming from outside the plane (or above the sheet of paper) that will pass through A but never intersect BC.
These lines are called skew lines. They are not parallel since they don’t exist in the same plane, but they never intersect, and there are infinitely many such skew lines for any line BC and point A.
Now, suppose I want to draw a line perpendicular to line BC. I can draw one in the plane that intersects it at point D, but in three-dimensional space, I can draw any number of lines passing through D which form a right angle with line AB. What does this mean? In the realm of 3D geometry, there are infinitely many lines that are perpendicular to a given line.
In 3D space, we cannot only draw lines, but we can also have entire planes next to each other, and just like lines, those planes can sometimes intersect. Two planes that intersect at right angles are perpendicular, and two planes that never intersect are parallel. The same parallel postulate holds for planes – given a plane A and a point B not on A, there is one and only one plane passing through B which never intersects A.
Let’s talk about another concept that is likely to appear on the test.
Planar Sections
Another major topic in 3D geometry is the concept of cross-sections. If you’ve ever enjoyed sliced cheese or a sandwich, or if you’ve opened a dollhouse to see the rooms inside, you’re familiar with the concept of a planar section. The slicing knife and the line where the dollhouse opens represent the “plane” in this case. By taking planar cuts of geometric solids, you can produce two-dimensional shapes. Here are a few examples of planar sections:
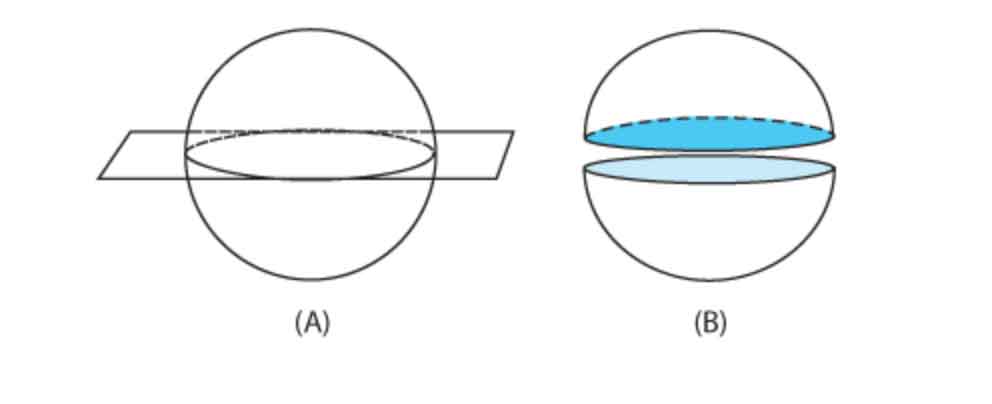
The image above depicts a sphere with a planar cut through it. Notice that the planar cut of this sphere produces a circle. In fact, no matter how you cut a sphere, the resulting shape will always be a circle; however, this isn’t always the case for other solids.
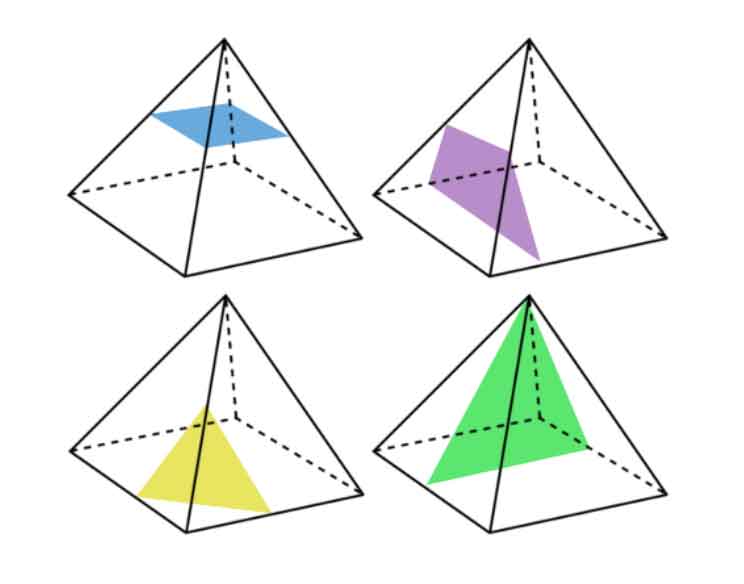
For example, take a look at this square pyramid above. We’ve taken four plane sections from it, and each one produces a different shape. Depending upon where you cut the pyramid, your planar section could be a square, a trapezoid, or a triangle.
Geometry: Transformational Geometry
Transformational geometry is the study of transforming shapes by means of various operations:
- Translation: Moving a shape without changing its orientation, like sliding a puck across the ice.
- Rotation: Turning a shape around a point, like spinning a record.
- Reflection: “Flipping” a shape over, like flipping a pancake.
- Dilation: Making a shape larger or smaller (but preserving proportions), like enlarging something in a magnifying glass.
Two shapes are said to be congruent if one can be obtained from the other by translation, reflection, and rotation. Two shapes are said to be similar if one can be obtained from the other by translation, reflection, rotation, and dilation.
The factor by which the length of every side of a dilated figure differs from the original is called the scale factor. For example, if the original figure contains a side AB, a similar figure will contain a side of length rAB, and the scale factor would be called r.
Geometry: Probability
Probability is the study of how likely an event is to occur. In probability terms, the word event refers to any outcome of a trial or experiment. For example, if you flip a coin, the coin landing on heads is one event, and the coin landing on tails is another. The probability of either event, for a single coin flip, is 50%, or 0.5.
The probability of an event is often expressed as a fraction or decimal. For an event A , we say that the probability that A will occur is P(A) , and P(A) is a number somewhere between 0 and 1, inclusive. An event with a probability of 0 is impossible – it will never occur. For example, the probability of rolling a 7 on a single six-sided die is 0. On the other hand, an event with a probability of 1, corresponding to 100%, is certain. On a regular coin, the probability of flipping either tails or heads is 100% – you’re guaranteed to get one or the other.
In the study of probability, it is important to think about how many outcomes are possible. For a given experiment, the set of all possible outcomes is called the sample space. For example, for rolling a single six-sided die, the possible outcomes are all the numbers from one to six, so the sample space for this experiment is S = {1,2,3,4,5,6}. The number of elements in the sample space is the number of possible outcomes in an experiment.
So, using this information, you can express the probability of a given outcome with the following ratio:
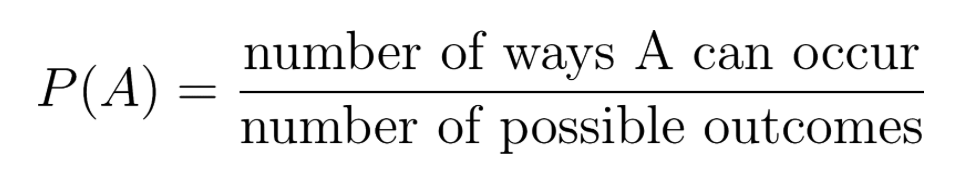
So, on a single six-sided die, what is the probability of rolling a 3? There is one way to roll a 3 and six possible outcomes, so P(3) = ⅙.
In this example, the probability for each outcome in the sample space is the same – ⅙. Think about this: What do you get if you add up all of the probabilities in the sample space? In this case, ⅙ + ⅙ + ⅙ + ⅙ + ⅙ + ⅙ = 1.
That’s not a coincidence. The probability distribution – that is, the set of all probabilities – for a sample space will always sum to one. This is true even if the probabilities within the sample space are different.
Let’s discuss a couple of concepts that will more than likely appear on the test.
Addition Rule for Disjoint Events
Think about rolling a six-sided die again. Is it possible to roll a four and a six at the same time? Not on a single die, it isn’t. These events are said to be mutually exclusive , which is also called disjoint. Here are some examples and nonexamples of disjoint events:
- Rolling a one or a three on a single six-sided die: DISJOINT.
- Rolling a two or rolling an even number: NOT DISJOINT (two is an even number, so if you roll a two, you’ve done both).
- Drawing a king or a queen from a deck of cards: DISJOINT.
- Drawing a king or drawing a heart: NOT DISJOINT (you may draw the king of hearts).
When two events are disjoint, we sometimes think about the probability of one thing or the other happening. The key word in this phrase is the word “OR.”
The probability that A or
B occurs is the sum of their individual probabilities:
P(A or B) = P(A) + P(B)
For example, suppose you spin a wheel to try to win a prize. There are 100 total slots on the wheel: 1 of those is green and wins a grand prize, 10 are yellow and win a second prize, 20 are blue and win a small prize, and the rest of the spaces are black and result in no win. What is the probability that you win the grand prize or a second prize?
P(Grand Prize OR Second Prize) = P(Grand Prize) + P(Second Prize)
= 1/100 + 10/100
= 11/100
= 0.11
Geometry: Statistics
Statistics is the study of data and how that data “looks.” A set of data points forms a “distribution” of that data, which can be visualized one of many ways including in histograms, stem and leaf plots, box plots, or two-way tables. One of the most basic concepts in statistics is the concept of the center of a distribution.
Center
The three measures of center are mean, median, and mode. The mean of a distribution is the sum of all data points, divided by n , the number of data points. This is also known as the average.
The median of a distribution is the value in the “middle” – as many above as below. In the case that n is even – that is, there are an even number of data points – there will not be a single value in the middle, but two. The average of these two data points is the median.
While mean and median are common measures of center, it is sometimes difficult to know which is better. The answer to this question is the concept of resistance. Suppose we poll a city block, asking each household their annual income. Suppose this block is mostly middle-class families making about $50,000 per year and one NBA superstar who makes millions. What do you think that NBA star will do to the mean? It will make it much higher than the average income for the rest of the families on the block; however, since the median doesn’t care how large or small the values above it may be, it is more or less unaffected by the extremely high income.
For this reason, we say that the median is resistant to outliers. An outlier is a data point that lies far outside the rest – it’s an oddball! If you suspect outliers in your data set, it is usually better to use the median than the mean.
The mode of a distribution is the value that appears most often in a set of data points. While mean and median deal with quantitative values – numbers, in other words – the mode is usually used in the case of categorical values. For example, if a survey asks, “what is your favorite flavor of ice cream?” it is not possible to find a mean or median for these responses – it is, however, possible to determine which flavor was mentioned most often, and that flavor is called the mode.
The Normal Distribution
The normal distribution is one of the most important concepts in statistics. It describes a distribution that is unimodal, meaning when you graph it in a histogram, it only has one “hump” and is symmetric and bell-shaped. This is a depiction of the normal distribution:
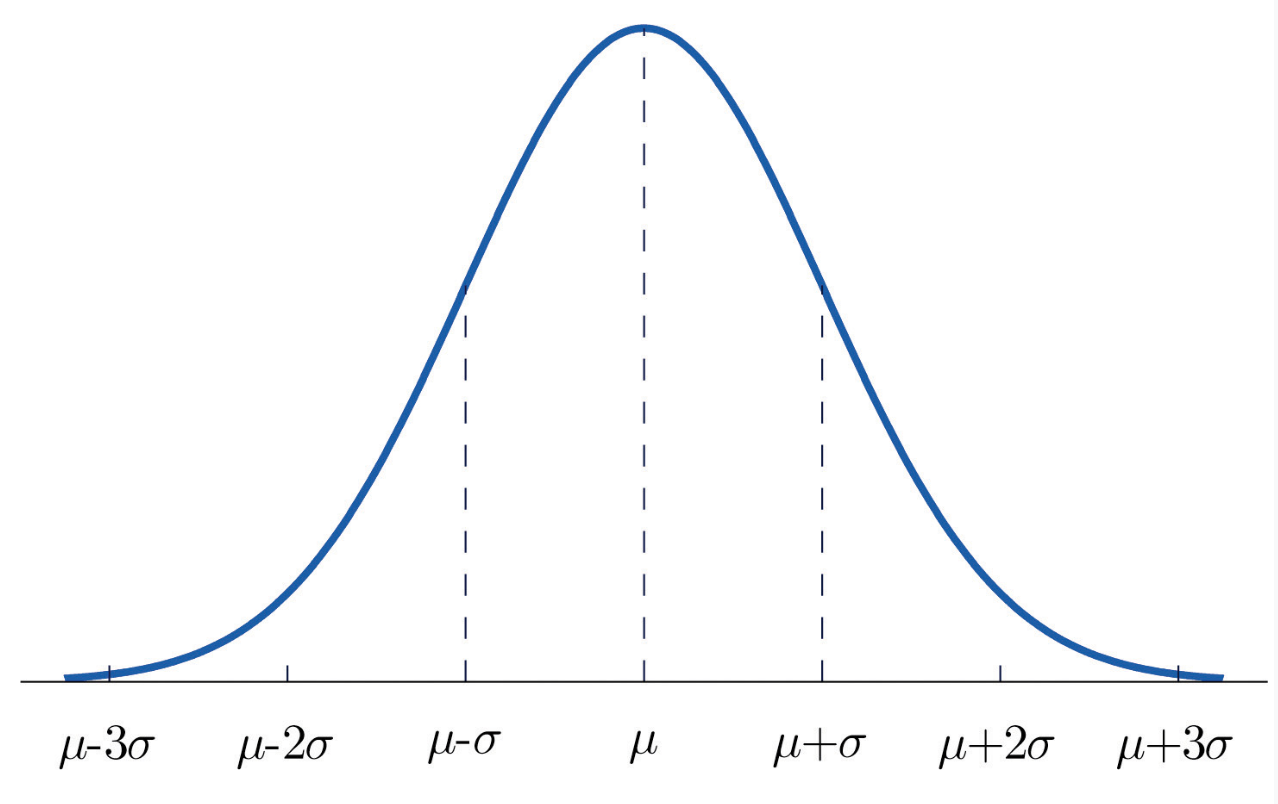
The normal distribution is centered at the mean, denoted here with the Greek letter μ, pronounced “mu,” and also uses the standard deviation, denoted here with the Greek letter σ, pronounced “sigma.”
The 68-95-99.7 Rule
This distribution leads to a very useful rule which appears often on tests as the 68-95-99.7 rule. This rule says the following:
- 68% of the data lies within one standard deviation of the mean.
- 95% of the data lies within two standard deviations of the mean.
- 99.7% of the data lies within three standard deviations of the mean.
Using these values as a starting point, we can further break down the normal distribution as follows:
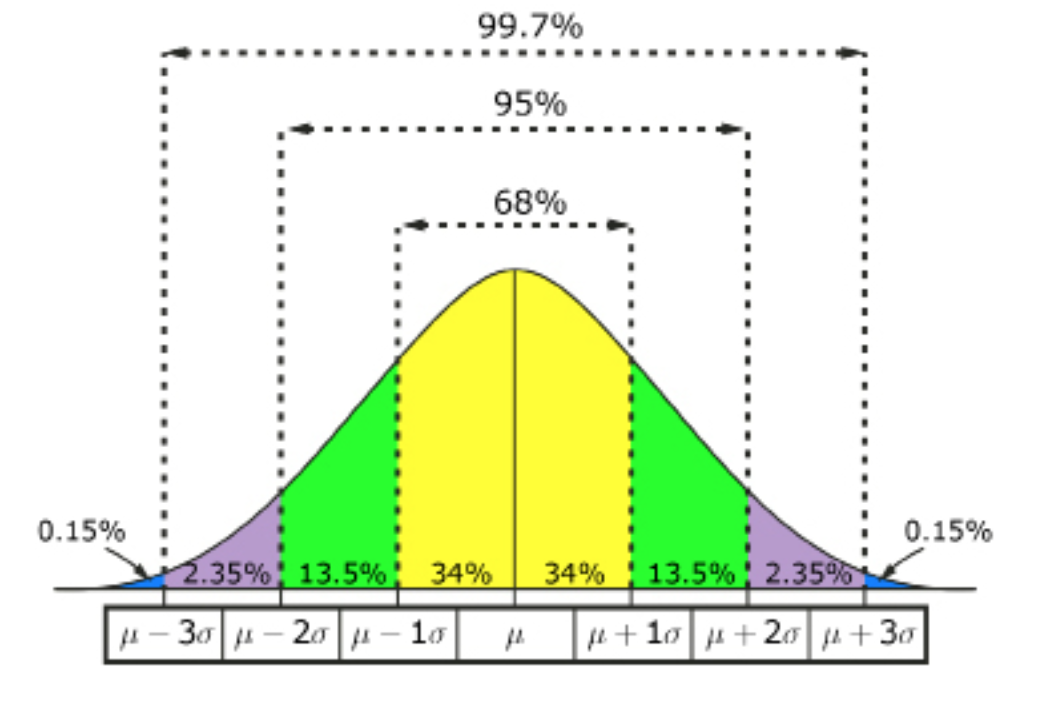
In other words, suppose that the heights of women are normally distributed with a mean height of 63.6 inches and a standard deviation of 2.5 inches. What percentage of women are taller than 68.6 inches?
If you think about it for a moment, you may realize that 68.6 is two “standard deviations” above the mean of 63.6 (because 63.6 + 2.5 + 2.5 = 68.3). That means that women who are taller will lie ABOVE the “μ+2σ” line on the normal distribution.
By looking at the graphic, you can see that there are 2.35% + 0.15% = 2.5% of women above that point – so the answer is that 2.5% of women are taller than 68.3 inches.
And that’s some basic info about the CSET Math Subtest II.
